
We multiply the numerators and multiply the denominators. Extension of this argument to the ratio of real numbers and their functions however, appears a bit trickier. To multiply rational expressions, we do just what we did with numerical fractions. It follows that the assumption is invalid, and p1/q1 divided by q2/p2 is defined for p2=0. This leads to a contradiction from the assumption above, since the product of 2 rational numbers in the form p1/q1 and p2/q2 does not exclude zero values for p1 and p2. Now given any 2 rational numbers in the form p1/q1 and p2/q2, their product is defined as p1p2/(q1q2) for integers p1, q1, p2, q2 except when q1≠0 or q2≠0.įurthermore, the division of any 2 rational numbers, p1/q1 divided by q2/p2 is defined as the product p1/q1 and p2/q2 = p1p2/(q1q2), although the product is undefined whenever q1≠0 or q2≠0. P1/q1 divided by q2/p2 is defined for p2=0.Īssume the contrary: p1/q1 divided by q2/p2 is not defined for p2=0. (x+1)/(x+2) divided by (x+3)/(x-4) … while the divisor might be undefined at x=4, is the quotient, really?Ĭonsider 2 rational numbers, p1/q1 and q2/p2, where p1, q1, p2, q2 are integers, q1≠0 and q2≠0, then:
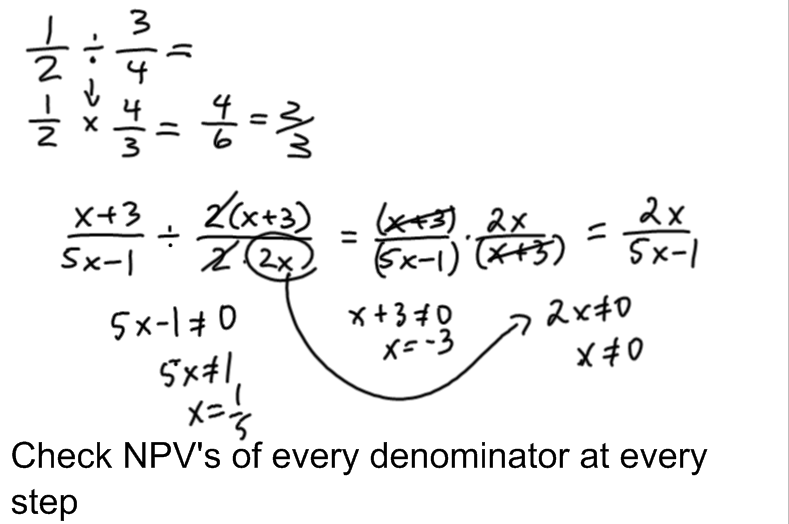
Perhaps that’s been formally established somewhere, but I’m not really convinced it holds. Is the result of the division really undefined when D=0? “To summarize, the expression that is the result of A/B divided by C/D is undefined when either B=0, C=0, or D=0.” Together you can come up with a plan to get you the help you need.= x 2 + x − 6 x 2 + 3 x − 10 ÷ x + 3 x − 5 = x 2 + x − 6 x 2 + 3 x − 10 ⋅ x − 5 x + 3 Multiply by the reciprocal = ( x + 3 ) ( x − 2 ) ( x + 5 ) ( x − 2 ) ⋅ x − 5 x + 3 Factor = ( x + 3 ) ( x − 2 ) ( x + 5 ) ( x − 2 ) ⋅ ( x − 5 ) x + 3 Cancel common factors = x − 5 x + 5 Multiply across \begin = x 2 + 3 x − 1 0 x 2 + x − 6 ÷ x − 5 x + 3 = x 2 + 3 x − 1 0 x 2 + x − 6 ⋅ x + 3 x − 5 = ( x + 5 ) ( x − 2 ) ( x + 3 ) ( x − 2 ) ⋅ x + 3 x − 5 = ( x + 5 ) ( x − 2 ) ( x + 3 ) ( x − 2 ) ⋅ x + 3 ( x − 5 ) = x + 5 x − 5 Multiply by the reciprocal Factor Cancel common factors Multiply across Find more Widget Gallery widgets in WolframAlpha. Multiplying rational expressions is similar to multiplying fractions. When we multiply two fractions, We multiply rational expressions using we10 divide out the common factors, e.g., 21 the same method.
#Multiplying and dividing rational expressions free
See your instructor as soon as possible to discuss your situation. Get the free 'Multiplying and Dividing Rational Expressions' widget for your website, blog, Wordpress, Blogger, or iGoogle. Multiplying and Dividing Rational Expressions (continued). MULTIPLY AND DIVIDE RATIONAL EXPRESSIONS WITH MONOMIALS Recall. You need to get help immediately or you will quickly be overwhelmed. Once we have canceled as many common factors as possible, we multiply numerators together and place the result over the product of the denominators. …no - I don’t get it! This is critical and you must not ignore it.

Is there a place on campus where math tutors are available? Can your study skills be improved? Step 2:Multiply the rational expressionsas shown above. Step 1:Write as multiplication of the reciprocal.
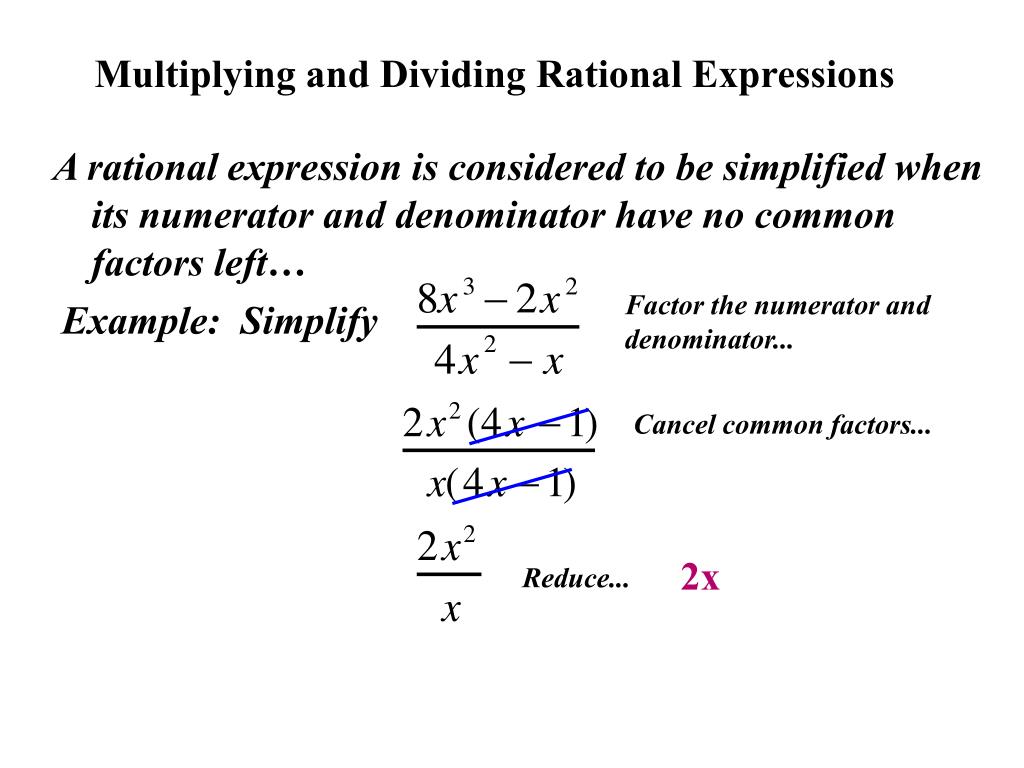
This is the fourth lesson in Algebra 2 Unit 8: Rational Functions. Dividing Rational Expressions where Q, S, and R do not equal 0. Who can you ask for help? Your fellow classmates and instructor are good resources. Color-coded and black and white-graphic organizers for simplifying, multiplying and dividing rational expressions. It is important to make sure you have a strong foundation before you move on. Math is sequential - every topic builds upon previous work. This must be addressed quickly as topics you do not master become potholes in your road to success. What did you do to become confident of your ability to do these things? Be specific! Congratulations! You have achieved your goals in this section! Reflect on the study skills you used so that you can continue to use them. I can.ĭetermine the values for which a rational expression is undefined. After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section. Simplify by dividing out common factors.Ī. Multiplying Rational Expressions Multiplying Dividing Purplemath With regular fractions, multiplying and dividing is fairly simple, and is much easier than adding and subtracting.Multiply the numerators and denominators together.Factor the numerators and denominators completely. Multiplying and dividing rational expressions works just like multiplying and dividing fractions.To multiply, first find the greatest common factors of the numerator and. Rewrite the division as the product of the first rational expression and the reciprocal of the second. Rational expressions are multiplied and divided the same way numeric fractions are.


 0 kommentar(er)
0 kommentar(er)
